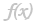ЕГЭ по Математике (профильный)
Решите неравенство $3^{2x+1}+4\cdot...
Задание:
Решите неравенство $3^{2x+1}+4\cdot 3^{x} + 2\cdot |3^{x}-2| \geq 5$.
Решение:
Введём обозначение $3^{x}=t$, $t > 0$. Неравенство примет вид $3t^{2}+4t+2|t-2|\geq 5$.
$\left[
\begin{gathered}
\left\{
\begin{gathered}
3t+4t+2t-4 \geq 5, \hfill
\\
t \geq 2; \hfill
\\
\end{gathered}
\right. \hfill
\\
\left\{
\begin{gathered}
3t^{2}+4t+2(-t+2)\geq 5, \hfill
\\
0 < t \leq 2; \hfill
\\
\end{gathered}
\right. \hfill
\\
\end{gathered}
\right.$
$\left[
\begin{gathered}
\left\{
\begin{gathered}
3t^{2}+6t-9\geq 0, \hfill
\\
t\geq 2; \hfill
\\
\end{gathered}
\right. \hfill
\\
\left\{
\begin{gathered}
3t^{2}+2t-1\geq 0, \hfill
\\
0< t\leq 2; \hfill
\\
\end{gathered}
\right. \hfill
\\
\end{gathered}
\right.$
$\left[
\begin{gathered}
\left\{
\begin{gathered}
t \leq -3; t \geq 1, \hfill
\\
t \geq 2; \hfill
\end{gathered}
\right. \hfill
\\
\left\{
\begin{gathered}
t \leq -1; t \geq \frac{1}{3}, \hfill
\\
0 < t \leq 2 \hfill
\\
\end{gathered}
\right. \hfill
\\
\end{gathered}
\right.$
$\left[
\begin{gathered}
t\geq 2, \\
\frac{1}{3} \leq t \leq 2; \\
\end{gathered}
\right.$ $t\geq \frac{1}{3}$.
$3^{x}\geq \frac{1}{3}$; $x \geq -1$.
Ответ: $[-1; +\infty)$.
$\left[
\begin{gathered}
\left\{
\begin{gathered}
3t+4t+2t-4 \geq 5, \hfill
\\
t \geq 2; \hfill
\\
\end{gathered}
\right. \hfill
\\
\left\{
\begin{gathered}
3t^{2}+4t+2(-t+2)\geq 5, \hfill
\\
0 < t \leq 2; \hfill
\\
\end{gathered}
\right. \hfill
\\
\end{gathered}
\right.$
$\left[
\begin{gathered}
\left\{
\begin{gathered}
3t^{2}+6t-9\geq 0, \hfill
\\
t\geq 2; \hfill
\\
\end{gathered}
\right. \hfill
\\
\left\{
\begin{gathered}
3t^{2}+2t-1\geq 0, \hfill
\\
0< t\leq 2; \hfill
\\
\end{gathered}
\right. \hfill
\\
\end{gathered}
\right.$
$\left[
\begin{gathered}
\left\{
\begin{gathered}
t \leq -3; t \geq 1, \hfill
\\
t \geq 2; \hfill
\end{gathered}
\right. \hfill
\\
\left\{
\begin{gathered}
t \leq -1; t \geq \frac{1}{3}, \hfill
\\
0 < t \leq 2 \hfill
\\
\end{gathered}
\right. \hfill
\\
\end{gathered}
\right.$
$\left[
\begin{gathered}
t\geq 2, \\
\frac{1}{3} \leq t \leq 2; \\
\end{gathered}
\right.$ $t\geq \frac{1}{3}$.
$3^{x}\geq \frac{1}{3}$; $x \geq -1$.
Ответ: $[-1; +\infty)$.
Задание добавил(а)
Создатель и главный администратор проекта ExamMe.
О задание:
Источник условия: Книга: Математика. Подготовка к ЕГЭ-2017. Профильный уровень. 40 тренировочных вариантов. Под редакцией Ф.Ф. Лысенко, С.Ю. Калабухова.
Источник решения: Книга: Решение заданий из книги указанной в источнике условия. Автор неизвестен.
Источник решения: Книга: Решение заданий из книги указанной в источнике условия. Автор неизвестен.
Обсуждения
Только зарегистрированные пользователи могут оставлять комментарии.